This list of open problems in representation stability is curated by Jeremy Miller and Peter Patzt. We first list three major conjectures that remained unsolved since the dawn of the subject of representation stability. Next, we give a longer list of open problems and questions that we have found in the literature. Finally, we link to six open problem lists from conferences on representation stability and related topics.

Major unsolved conjectures in Representation Stability

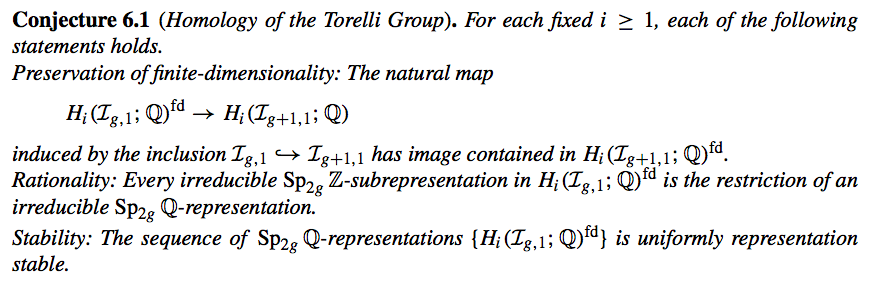
M1) The following conjecture by Church and Farb asserts representation stability for the Torelli groups. A similar conjecture is made for the Torelli subgroups of the automorphism groups of free groups.

Relevant literature:

• Dennis Johnson, The structure of the Torelli group III: The abelianization of I, Topology 24 (1985), no. 2, 127–144.
• Søren K. Boldsen and Mia Hauge Dollerup, Towards representation stability for the second homology of the Torelli group, Geom. Topol. 16 (2012), no. 3, 1725–1765
• Church, Thomas; Farb, Benson, Representation theory and homological stability, Adv. Math. 245 (2013), 250–314.
• Thomas Church, Jordan S. Ellenberg, and Benson Farb, FI–modules and stability for representations of symmetric groups, Duke Math. J. 164 (2015), no. 9, 1833–1910.
• Matthew Day and Andrew Putman, On the second homology group of the Torelli subgroup of Aut(F_n), Geometry & Topology 21 (2017), no. 5, 2851–2896.
• Jeremy Miller, Peter Patzt, Jennifer C.H. Wilson, Central stability for the homology of congruence subgroups and the second homology of Torelli groups, Adv. Math., 354 (2019), 106740.

M2) This conjecture by Church, Farb, and Putman asserts the vanishing of the cohomology of special linear groups near its virtual cohomological dimension. 

Relevant literature:

• Ronnie Lee and R. H. Szczarba, On the homology and cohomology of congruence subgroups, Invent. Math. 33 (1976), no. 1, 15–5.
• Thomas Church, Benson Farb, and Andrew Putman, A stability conjecture for the unstable cohomology of SL_n Z, mapping class groups, and Aut(F_n), Algebraic topology: applications and new directions, Contemp. Math., vol. 620, Amer. Math. Soc., Providence, RI, 2014, pp. 55–70.
• Thomas Church and Andrew Putman, The codimension-one cohomology of SL_n Z, Geom. Topol. 21 (2017), no. 2, 999–1032.
• Avner Ash, Andrew Putman, and Steven V. Sam, Homological vanishing for the Steinberg representation, Compos. Math. 154 (2018), no. 6, 1111–1130.
• Jeremy Miller, Rohit Nagpal, Peter Patzt, Stability in the high-dimensional cohomology of congruence subgroups, Compos. Math. 156(4) (2020), 822-861.

M3) This conjecture by Sam and Snowden asserts that all finitely generated TCA’s are noetherian. 

Relevant literature:

• Steven V Sam, Andrew Snowden. Stability patterns in representation theory. Forum Math. Sigma 3 (2015), e11, 108 pp.
• Rohit Nagpal, Steven V Sam, Andrew Snowden. Noetherianity of some degree two twisted commutative algebras. Selecta Math. (N.S.) 22 (2016), no. 2, 913–937.
• Jan Draisma, Topological Noetherianity of polynomial functors, J. Am. Math. Soc. 32(3), 691-707 (2019).
• Rob H. Eggermont, Andrew Snowden, Topological noetherianity for algebraic representations of infinite rank classical groups, preprint

More open problems in representation stability

Classical representation stability

• Church-Farb https://arxiv.org/abs/1008.1368, Section 6
  Note: See inequivalent reformulation in terms of VIC(Z) and SI(Z) modules in Miller-Patzt-Wilson https://arxiv.org/pdf/1704.04449.pdf
• Church-Ellenberg-Farb https://arxiv.org/pdf/1204.4533.pdf, Problems 1.10, 1.12, 4.1b
• Farb https://arxiv.org/pdf/1404.4065.pdf, Problems 2.1, 3.5, 4.7, 6.2, 7.1
  Note: Problem 7.1(3) is false for GL_n(Z) by Putman-Sam.

Improved ranges and secondary stability

• Miller-Wilson https://arxiv.org/pdf/1611.01920.pdf, Section 3.6
  Note: Bibby-Gadish https://arxiv.org/abs/1911.02125 and Ho https://quoc-ho.github.io/research/papers/higher_rep_stab_conf.pdf have related theorems.
• Sah https://www.sciencedirect.com/science/article/pii/0022404989900613, Section 4, in particular Problem 4.10, a conjecture of improved ranges for classical groups by Milnor
  Note: These were partly resolved by Galatius-Kupers-Randal-Williams https://arxiv.org/pdf/2005.05620.pdf and Sprehn-Wahl https://arxiv.org/pdf/1812.08742.pdf
• Galatius-Kupers-Randal-Williams https://arxiv.org/pdf/2005.05620.pdf, Conj 7.7

Arithmetic statistics

• Vakil-Wood https://arxiv.org/pdf/1208.3166.pdf, many conjectures in the introduction.
  Note:
  Kupers-Miller-Tran https://arxiv.org/pdf/1410.5497.pdf proved Conj F.
  Banerjee https://arxiv.org/abs/2003.13916 resolved Conj G’ and H’

High dimensional cohomology of arithmetic groups

• Kupers-Miller-Patzt-Wilson https://arxiv.org/pdf/2006.10906.pdf, Section 5
• Miller-Nagpal-Patzt https://arxiv.org/pdf/1806.11131.pdf, Section 6.1
• Church-Farb-Putman https://arxiv.org/pdf/1208.3216.pdf, many more conjectures than M2.

Representations of the symmetric groups

• Nagpal https://arxiv.org/pdf/1810.04592.pdf, Question 1.5, 1.9, 1.14
• Nagpal-Snowden https://arxiv.org/pdf/1705.10028.pdf, Section 1.6
• Rohit’s thesis https://arxiv.org/pdf/1505.04294.pdf, Section 5
• Sam-Snowden https://arxiv.org/pdf/2006.04328.pdf, Section 1.5
• Sam-Snowden https://arxiv.org/pdf/1705.10718.pdf, Section 1.2
• Sam-Snowden https://arxiv.org/pdf/1302.5859.pdf, Section 8

Graphs

• Pawlowski-Ramos-Rhoades https://arxiv.org/pdf/1907.07268.pdf, Section 4.
• Ramos-White https://arxiv.org/pdf/1810.08475.pdf, Section 1.5.
• Ramos https://arxiv.org/pdf/1805.05316.pdf, Rem 1.1 asks if, for finitely generated FI-graphs G_n, the FI-modules H_i(UConf_m(G_n)) (for fixed i and m) are finitely generated.
• Ramos https://arxiv.org/pdf/1609.05611.pdf Section 4.
  Note: Conj 4.3 has been proved by An-Drummond-Cole-Knudsen.
• Ramos https://arxiv.org/pdf/1606.02673.pdf, A question about FI_d-modules between Thm A and B.

Problem sessions

AIM Workshop 2016: Representation Stability

Copenhagen Masterclass 2017: Cohomology of arithmetic groups

Oberwolfach workshop 2018: Topology of Arrangements and Representation Stability (Problem session p113)

Midwest Representation Stability Research Meeting 2019

PIMS/NSF Workshop 2019: Arithmetic Topology

AMS Sectional 2020 (Online): Stability in Topology, Arithmetic, and Representation theory